Relative Maximum Vs Absolute Maximum

Show Mobile Notice Show All NotesHide All Notes

Mobile Observe

You appear to be on a device with a "narrow" screen width (i.east. you are probably on a mobile phone). Due to the nature of the mathematics on this site it is best views in mural mode. If your device is not in landscape mode many of the equations will run off the side of your device (should be able to scroll to come across them) and some of the menu items will be cut off due to the narrow screen width.

Section 4-3 : Minimum and Maximum Values

Many of our applications in this affiliate will revolve around minimum and maximum values of a function. While we can all visualize the minimum and maximum values of a function we want to be a little more specific in our work here. In detail, nosotros desire to differentiate betwixt 2 types of minimum or maximum values. The following definition gives the types of minimums and/or maximums values that we'll be looking at.

Definition

We say that $f\left( x \right)$ has an absolute (or global) maximum at $x = c$ if$f\left( x \right) \le f\left( c \right)$ for every $ten$ in the domain nosotros are working on.
We say that $f\left( x \right)$ has a relative (or local) maximum at $x = c$ if $f\left( x \right) \le f\left( c \right)$ for every $10$ in some open interval around $10 = c$.
We say that $f\left( x \right)$ has an absolute (or global) minimum at $x = c$ if $f\left( 10 \right) \ge f\left( c \correct)$ for every $ten$ in the domain nosotros are working on.
We say that $f\left( x \right)$ has a relative (or local) minimum at $x = c$ if$f\left( ten \right) \ge f\left( c \right)$ for every $x$ in some open interval around $x = c$.

Note that when nosotros say an "open up interval effectually$10 = c$" nosotros mean that we can find some interval $\left( {a,b} \right)$, not including the endpoints, such that $a < c < b$. Or, in other words, $c$ will be contained somewhere inside the interval and will not be either of the endpoints.

Likewise, we volition collectively telephone call the minimum and maximum points of a function the extrema of the part. So, relative extrema volition refer to the relative minimums and maximums while absolute extrema refer to the absolute minimums and maximums.

Now, let'due south talk a little bit virtually the subtle departure between the absolute and relative in the definition above.

We will have an accented maximum (or minimum) at $x = c$ provided $f\left( c \right)$ is the largest (or smallest) value that the role volition ever have on the domain that we are working on. Too, when nosotros say the "domain nosotros are working on" this only means the range of $ten$'s that nosotros have chosen to work with for a given trouble. In that location may be other values of $x$ that nosotros can actually plug into the function but take excluded them for some reason.

A relative maximum or minimum is slightly different. All that'due south required for a point to be a relative maximum or minimum is for that bespeak to exist a maximum or minimum in some interval of $ten$'south effectually $x = c$. There may be larger or smaller values of the part at some other place, only relative to $x = c$, or local to $x = c$, $f\left( c \right)$ is larger or smaller than all the other function values that are near it.

Note also that in society for a point to be a relative extrema nosotros must be able to expect at part values on both sides of $x = c$ to come across if it actually is a maximum or minimum at that point. This means that relative extrema do not occur at the end points of a domain. They can but occur interior to the domain.

There is really some contend on the preceding point. Some folks do feel that relative extrema tin occur on the terminate points of a domain. However, in this class nosotros will exist using the definition that says that they tin can't occur at the end points of a domain. This volition be discussed in a little more detail at the stop of the department one time we have a relevant fact taken care of.

It's ordinarily easier to get a feel for the definitions by taking a quick look at a graph.

This is a sketch of some unknown function. The left most point is in the third quadrant and marked as

For the function shown in this graph we have relative maximums at $x = b$ and $x = d$. Both of these points are relative maximums since they are interior to the domain shown and are the largest betoken on the graph in some interval around the point. Nosotros besides have a relative minimum at $x = c$ since this point is interior to the domain and is the everyman point on the graph in an interval effectually it. The far-right end bespeak, $x = e$, volition not be a relative minimum since it is an finish point.

The role will have an absolute maximum at $x = d$ and an absolute minimum at $10 = a$. These ii points are the largest and smallest that the role will ever be. We can also detect that the absolute extrema for a part will occur at either the endpoints of the domain or at relative extrema. Nosotros will employ this idea in later on sections and then information technology'southward more important than it might seem at the present time.

Let's take a quick look at some examples to make sure that nosotros have the definitions of accented extrema and relative extrema straight.

Example 1 Identify the absolute extrema and relative extrema for the following function. \[f\left( 10 \right) = {ten^2}\hspace{0.25in}{\mbox{on}}\hspace{0.25in}\left[ { - 1,2} \right]\]

Bear witness Solution

Since this function is easy enough to graph let's do that. However, we but desire the graph on the interval $\left[ { - 1,2} \correct]$. Here is the graph,

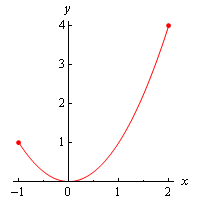
$Graph of $f\left( x \right)={{x}^{2}}$ on the domain $-1 \le x \le 2$ with dots at the (-1,1) and (2,4) to indicate that the graph does not extend past these two points.$

Note that nosotros used dots at the end of the graph to remind us that the graph ends at these points.

We tin now identify the extrema from the graph. It looks like we've got a relative and absolute minimum of aught at $10 = 0$ and an absolute maximum of iv at $x = two$. Note that $x = - 1$ is not a relative maximum since it is at the end point of the interval.

This part doesn't have any relative maximums.

Equally nosotros saw in the previous example functions do not have to have relative extrema. It is completely possible for a role to not have a relative maximum and/or a relative minimum.

Case 2 Identify the accented extrema and relative extrema for the post-obit function. \[f\left( 10 \right) = {x^2}\hspace{0.25in}{\mbox{on}}\hspace{0.25in}\left[ { - 2,2} \correct]\]

Show Solution

Hither is the graph for this function.

$Graph of $f\left( x \right)={{x}^{2}}$ on the domain $-2 \le x \le 2$ with dots at the (-2,4) and (2,4) to indicate that the graph does not extend past these two points.$

In this case we notwithstanding have a relative and absolute minimum of zero at $x = 0$. We likewise nonetheless have an absolute maximum of 4. However, unlike the get-go example this will occur at two points, $x = - ii$ and $x = 2$.

Again, the function doesn't accept whatever relative maximums.

As this example has shown there can but be a single absolute maximum or accented minimum value, just they can occur at more than than one identify in the domain.

Case 3 Identify the absolute extrema and relative extrema for the following part. \[f\left( x \right) = {10^2}\]

Show Solution

In this case we've given no domain and so the supposition is that nosotros volition take the largest possible domain. For this function that means all the real numbers. Here is the graph.

$Graph of $f\left( x \right)={{x}^{2}}$ with no domain specified and arrows at the ends pointing upwards to indicate the graph continues.$

In this example the graph doesn't end increasing at either cease and then there are no maximums of any kind for this function. No affair which point we pick on the graph there will be points both larger and smaller than it on either side so we can't have any maximums (of any kind, relative or accented) in a graph.

Nosotros yet have a relative and absolute minimum value of zero at $x = 0$.

So, some graphs tin have minimums but not maximums. Likewise, a graph could take maximums but not minimums.

Instance 4 Identify the absolute extrema and relative extrema for the post-obit function. \[f\left( x \right) = {x^3}\hspace{0.25in}{\mbox{on}}\hspace{0.25in}\left[ { - ii,2} \right]\]

Bear witness Solution

Here is the graph for this function.

$Graph of $f\left( x \right)={{x}^{3}}$ on the domain $-2 \le x \le 2$ with dots at the (-2,-8) and (2,8) to indicate that the graph does not extend past these two points.$

This function has an absolute maximum of eight at $x = 2$ and an absolute minimum of negative eight at $ten = - ii$. This role has no relative extrema.

And then, a function doesn't have to have relative extrema as this example has shown.

Instance 5 Place the absolute extrema and relative extrema for the following function. \[f\left( ten \right) = {10^iii}\]

Show Solution

Once more, we aren't restricting the domain this fourth dimension then hither's the graph.

$Graph of $f\left( x \right)={{x}^{3}}$ with no domain specified and arrows at the ends to indicate the graph continues.$

In this instance the function has no relative extrema and no absolute extrema.

Equally we've seen in the previous case functions don't have to have whatsoever kind of extrema, relative or absolute.

Case half-dozen Place the absolute extrema and relative extrema for the following role. \[f\left( 10 \correct) = \cos \left( x \right)\]

Show Solution

Nosotros've non restricted the domain for this function. Hither is the graph.

$Graph of $f\left( x \right)=\cos \left( x \right)$ on the domain $\left[ -3\pi ,3\pi \right]$ and arrows at the ends to indicate the graph continues.$

Cosine has extrema (relative and absolute) that occur at many points. Cosine has both relative and absolute maximums of 1 at

\[x = \ldots - 4\pi ,\, - ii\pi ,\,\,0,\,\,2\pi ,\,\,4\pi , \ldots \]

Cosine also has both relative and accented minimums of -1 at

\[x = \ldots - 3\pi ,\, - \pi ,\,\,\pi ,\,\,3\pi , \ldots \]

As this case has shown a graph can in fact have extrema occurring at a large number (infinite in this case) of points.

We've at present worked quite a few examples and nosotros tin can use these examples to come across a nice fact virtually absolute extrema. Commencement allow'southward notice that all the functions above were continuous functions. Next notice that every time nosotros restricted the domain to a closed interval (i.e. the interval contains its end points) we got absolute maximums and absolute minimums. Finally, in only one of the three examples in which nosotros did not restrict the domain did we go both an absolute maximum and an accented minimum.

These observations lead us the following theorem.

Extreme Value Theorem

Suppose that $f\left( x \correct)$ is continuous on the interval $\left[ {a,b} \correct]$ so at that place are two numbers $a \le c,d \le b$ then that $f\left( c \right)$ is an absolute maximum for the role and $f\left( d \right)$ is an absolute minimum for the function.

So, if we have a continuous function on an interval $\left[ {a,b} \correct]$ then we are guaranteed to have both an absolute maximum and an absolute minimum for the function somewhere in the interval. The theorem doesn't tell usa where they volition occur or if they will occur more once, only at to the lowest degree it tells united states that they do be somewhere. Sometimes, all that nosotros need to know is that they do exist.

This theorem doesn't say anything nigh absolute extrema if we aren't working on an interval. Nosotros saw examples of functions above that had both accented extrema, one absolute extrema, and no absolute extrema when we didn't restrict ourselves downwards to an interval.

The requirement that a function be continuous is likewise required in order for us to use the theorem. Consider the instance of

\[f\left( x \right) = \frac{ane}{{{ten^2}}}\hspace{0.25in}{\mbox{on}}\hspace{0.25in}[ - 1,1]\]

Here's the graph.

$Graph of $f\left( x \right)=\frac{1}{{{x}^{2}}}$ on the domain $-1 \le x \le 1$ with dots at the (-1,1) and (1,1) to indicate that the graph does not extend past these two points. There is also a gap as the graph approaches the y-axis to indicate that the graph will continue to move up the y-axis as it approaches.$

This part is not continuous at $x = 0$ equally we motion in towards zero the function is budgeted infinity. And so, the function does not accept an absolute maximum. Note that it does accept an absolute minimum however. In fact the absolute minimum occurs twice at both $ten = - i$ and $x = one$.

If we changed the interval a picayune to say,

\[f\left( x \right) = \frac{1}{{{10^2}}}\hspace{0.25in}{\mbox{on}}\hspace{0.25in}\left[ {\frac{ane}{2},i} \right]\]

the function would now have both accented extrema. We may simply run into problems if the interval contains the point of aperture. If it doesn't then the theorem will concur.

Nosotros should also indicate out that just because a office is not continuous at a point that doesn't hateful that it won't have both absolute extrema in an interval that contains that indicate. Below is the graph of a function that is not continuous at a bespeak in the given interval and yet has both accented extrema.

This is the graph of some unknown function. The left most point is in the third quadrant and labeled

This graph is not continuous at $x = c$, all the same it does have both an absolute maximum ($x = b$) and an absolute minimum ($x = c$). Also note that, in this case one of the accented extrema occurred at the point of discontinuity, but it doesn't need to. The absolute minimum could simply have easily been at the other end point or at some other point interior to the region. The point here is that this graph is not continuous and nonetheless does have both absolute extrema

The point of all this is that we demand to be careful to merely employ the Extreme Value Theorem when the conditions of the theorem are met and not misinterpret the results if the conditions aren't met.

In order to employ the Extreme Value Theorem we must accept an interval that includes its endpoints, frequently called a closed interval, and the office must be continuous on that interval. If we don't have a closed interval and/or the function isn't continuous on the interval then the function may or may not have accented extrema.

We need to discuss 1 terminal topic in this section earlier moving on to the first major application of the derivative that we're going to be looking at in this chapter.

Fermat's Theorem

If $f\left( x \right)$ has a relative extrema at $x = c$ and $f'\left( c \right)$ exists and then $x = c$ is a critical indicate of $f\left( x \right)$. In fact, it will be a critical point such that $f'\left( c \right) = 0$.

To see the proof of this theorem see the Proofs From Derivative Applications section of the Extras chapter.

Besides annotation that nosotros can say that $f'\left( c \right) = 0$ because we are likewise bold that $f'\left( c \correct)$ exists.

This theorem tells us that there is a dainty relationship between relative extrema and critical points. In fact, information technology will let u.s. to get a list of all possible relative extrema. Since a relative extrema must be a critical point the list of all disquisitional points will requite the states a list of all possible relative extrema.

Consider the case of $f\left( 10 \right) = {x^ii}$. We saw that this function had a relative minimum at $ten = 0$ in several earlier examples. So according to Fermat's theorem $ten = 0$ should be a critical point. The derivative of the role is,

\[f'\left( x \correct) = 2x\]

Sure plenty $x = 0$ is a disquisitional signal.

Be conscientious not to misuse this theorem. It doesn't say that a critical point will exist a relative extrema. To see this, consider the following instance.

\[f\left( 10 \right) = {x^iii}\hspace{0.25in}\hspace{0.25in}f'\left( ten \right) = iii{x^2}\]

Clearly $10 = 0$ is a disquisitional point. However, nosotros saw in an before case this part has no relative extrema of any kind. So, critical points do not accept to exist relative extrema.

Besides annotation that this theorem says nada about absolute extrema. An absolute extrema may or may not be a critical signal.

Before nosotros leave this section we demand to hash out a couple of bug.

First, Fermat's Theorem only works for critical points in which $f'\left( c \right) = 0$. This does not, however, mean that relative extrema won't occur at critical points where the derivative does not exist. To see this consider $f\left( 10 \right) = \left| x \right|$. This role clearly has a relative minimum at $10 = 0$ and nonetheless in a previous department we showed in an example that $f'\left( 0 \right)$ does not exist.

What this all means is that if we desire to locate relative extrema all we really need to do is look at the disquisitional points every bit those are the places where relative extrema may exist.

Finally, recall that at that outset of the section we stated that relative extrema will not exist at endpoints of the interval we are looking at. The reason for this is that if we allowed relative extrema to occur there it may well (and in fact most of the time) violate Fermat's Theorem. There is no reason to expect end points of intervals to exist disquisitional points of any kind. Therefore, we do not allow relative extrema to be at the endpoints of intervals.